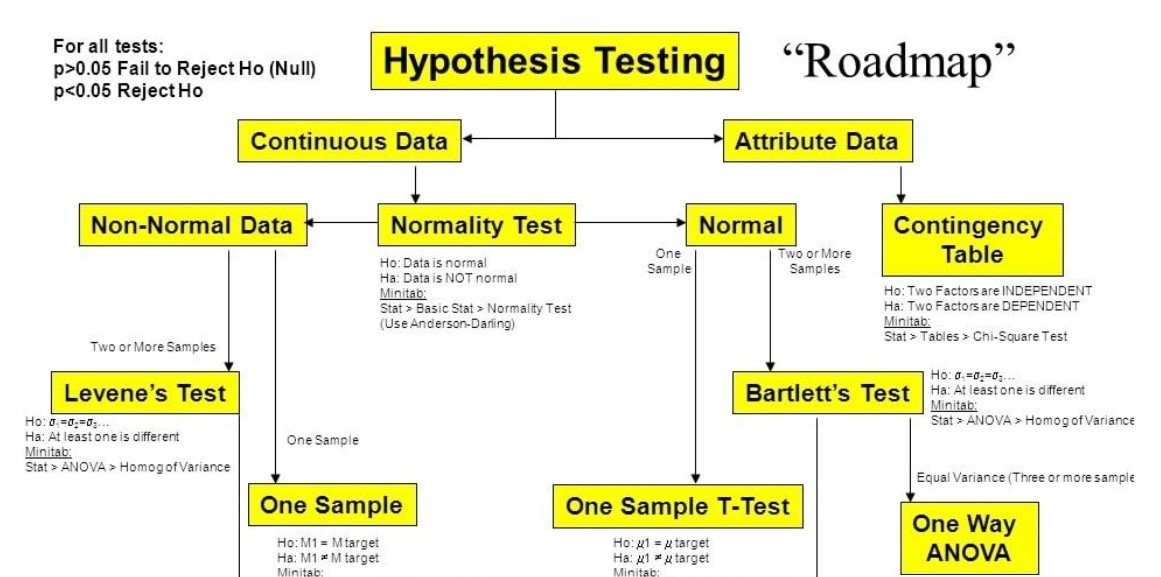
Hypothesis Testing
Hypothesis testing is a statistical method used to make inferences and draw conclusions about a population based on sample data. It involves formulating a hypothesis, collecting data, and analyzing the data to determine if the evidence supports or contradicts the hypothesis.
Hypothesis testing follows a structured process with defined steps.
Here's a general outline of hypothesis testing: Formulating the Hypotheses:
Null Hypothesis (H0): It states the assumption or claim to be tested. It is typically the hypothesis of no effect or no difference.
Alternative Hypothesis (Ha or H1): It represents the alternative claim or effect that is being tested. It contradicts the null hypothesis.
Selecting the Significance Level (α): The significance level (α) is the predetermined threshold used to determine the strength of evidence required to reject the null hypothesis.
Commonly used significance levels are 0.05 (5%) and 0.01 (1%).
These values indicate the probability of obtaining the observed result by chance if the null hypothesis is true.
Collecting and Analyzing the Data:
Data is collected through appropriate sampling methods. Descriptive statistics and appropriate statistical tests are used to analyze the data, depending on the nature of the research question and the type of data.
Calculating the Test Statistic
The test statistic is a value calculated from the sample data that helps assess the likelihood of observing the data under the null hypothesis. The choice of test statistic depends on the type of data and the hypothesis being tested.
Determining the Critical Region and P-Value
The critical region is the range of test statistic values that leads to the rejection of the null hypothesis. The p-value is the probability of obtaining a test statistic as extreme as, or more extreme than, the observed value, assuming the null hypothesis is true.
Making a Decision
If the test statistic falls within the critical region or the p-value is smaller than the significance level, the null hypothesis is rejected. If the test statistic does not fall within the critical region or the p-value is greater than the significance level, there is insufficient evidence to reject the null hypothesis.
Drawing Conclusions
Based on the decision made in step 6, conclusions are drawn about the hypothesis being tested and its implications for the population.
Hypothesis testing is widely used in various fields to evaluate research questions, compare groups, test relationships, and validate assumptions. It helps researchers make informed decisions and draw meaningful conclusions based on statistical evidence.